Angles

Angles are a fundamental concept in geometry. They are formed when two rays meet at a single point, called the vertex.

Definitions

Definition Angle

An angle is the measure of rotation between two rays that share a common endpoint, called the vertex.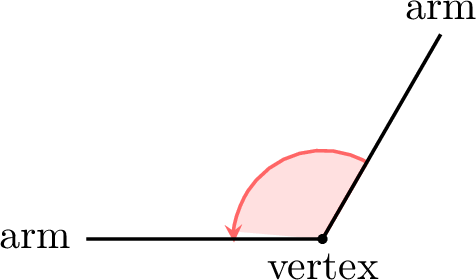

Definition Naming Angles with Three Points

An angle is named using three points with the symbol $\Angle{ABC}$, where $B$ is the vertex of the angle, and $A$ and $C$ are points on the two sides of the angle. The vertex $B$ is always written in the middle to indicate the angle’s center.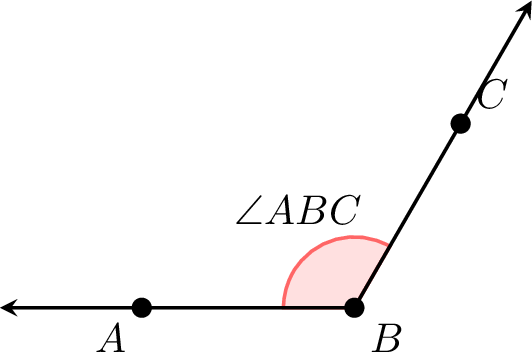

Degrees

A full turn, or complete circle, is divided into 360 equal parts called degrees, a convention established by ancient Babylonian astronomers.

Definition Degree Unit

A degree is a unit of angle measurement. A full turn measures $360^\circ$.

Definition Measure of an Angle in Degrees

The measure of an angle in degrees is the fraction of a full turn it represents.

Example

Calculate the measure of an angle that represents one-third of a full turn.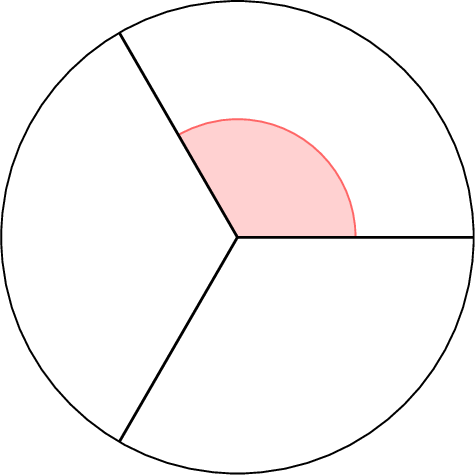

Answer

$$\begin{aligned}\text{Angle} &= \frac{1}{3} \text{ of } 360^\circ \\&= 360^\circ \div 3 \\&= 120^\circ\end{aligned}$$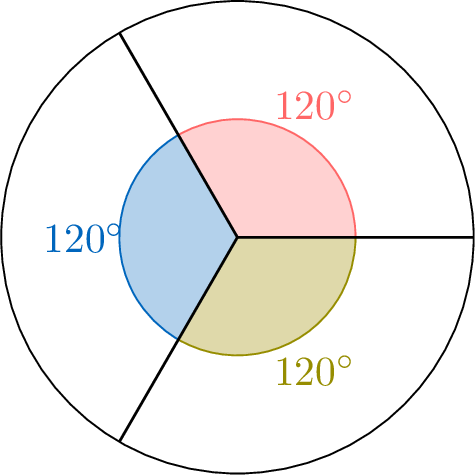

Measuring and Drawing Angles with a Protractor

Definition Protractor

A protractor is a tool used to measure and draw angles in degrees. It is typically a semi-circular tool with a scale marked from $0^\circ$ to $180^\circ$.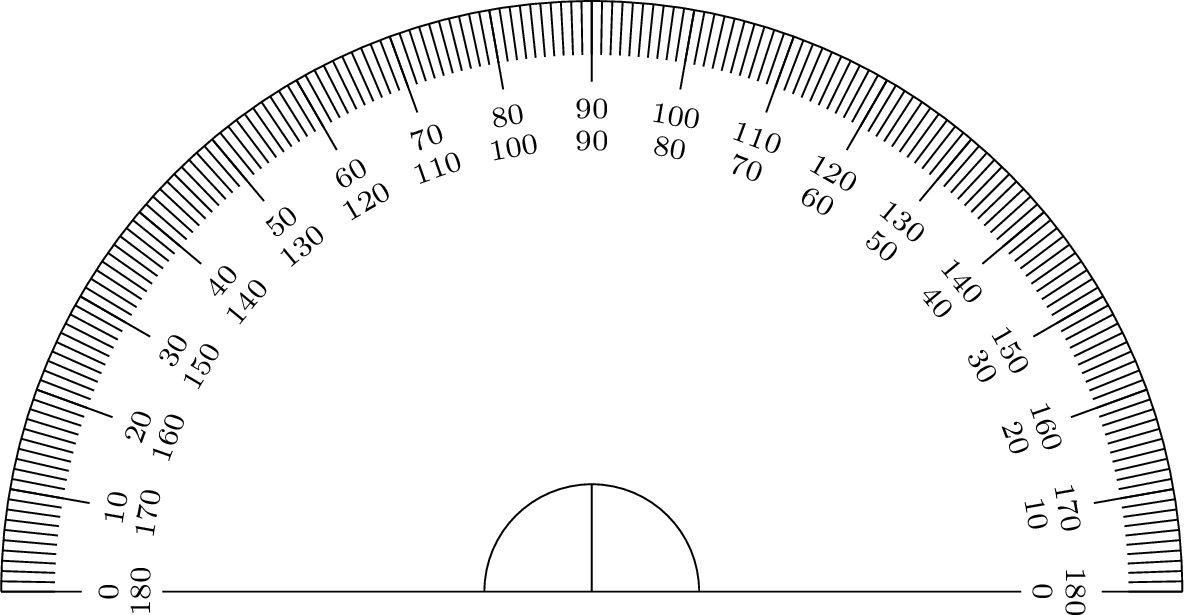

Method Measuring an Angle with a Protractor

Place the protractor’s origin (center point) over the vertex of the angle.
Align one ray of the angle with the protractor’s baseline (the $0^\circ$ mark).
Observe where the other ray intersects the protractor’s scale.
Read the angle measure in degrees from the scale.

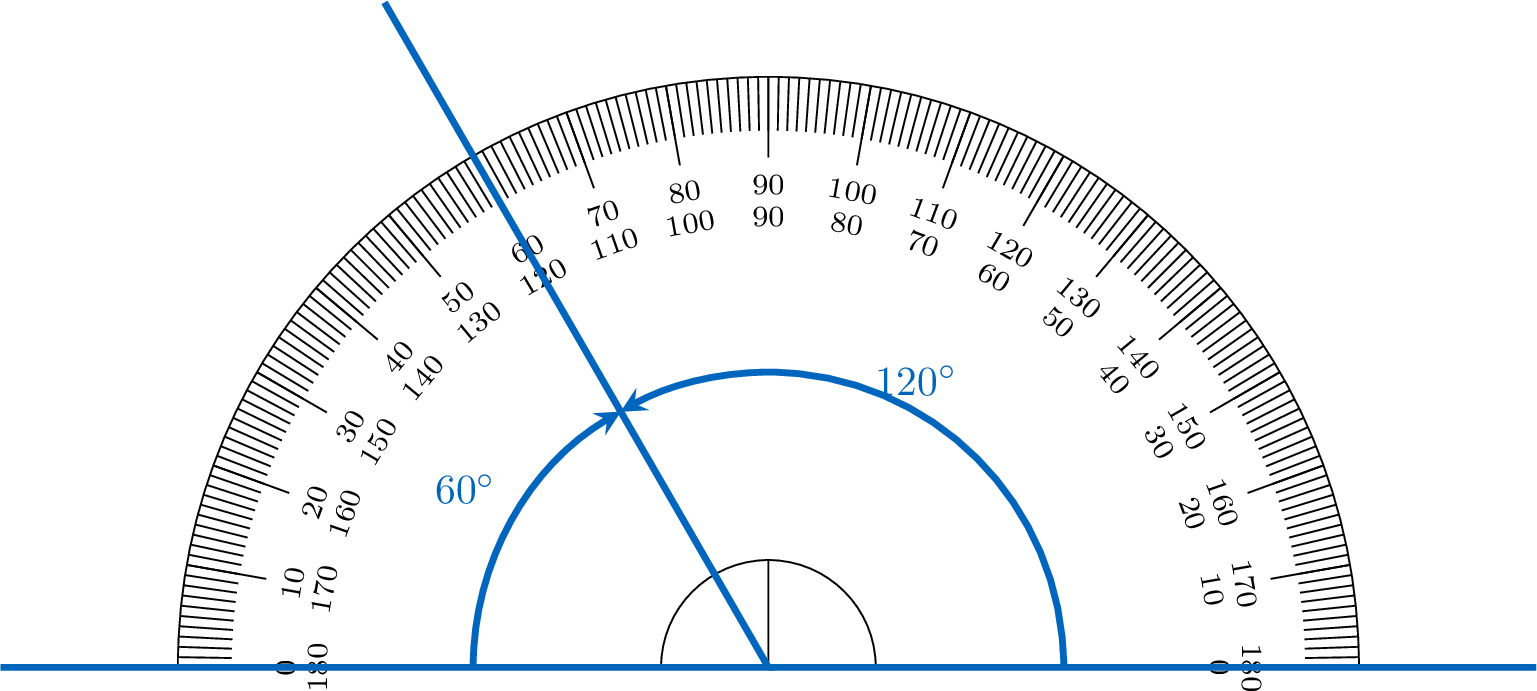
Example

Measure the following angle.

Answer

The angle measures $140^\circ$.

Method Drawing an Angle with a Protractor

Draw a ray starting from a point (the vertex).
Place the protractor’s origin over the vertex and align the baseline with the ray.
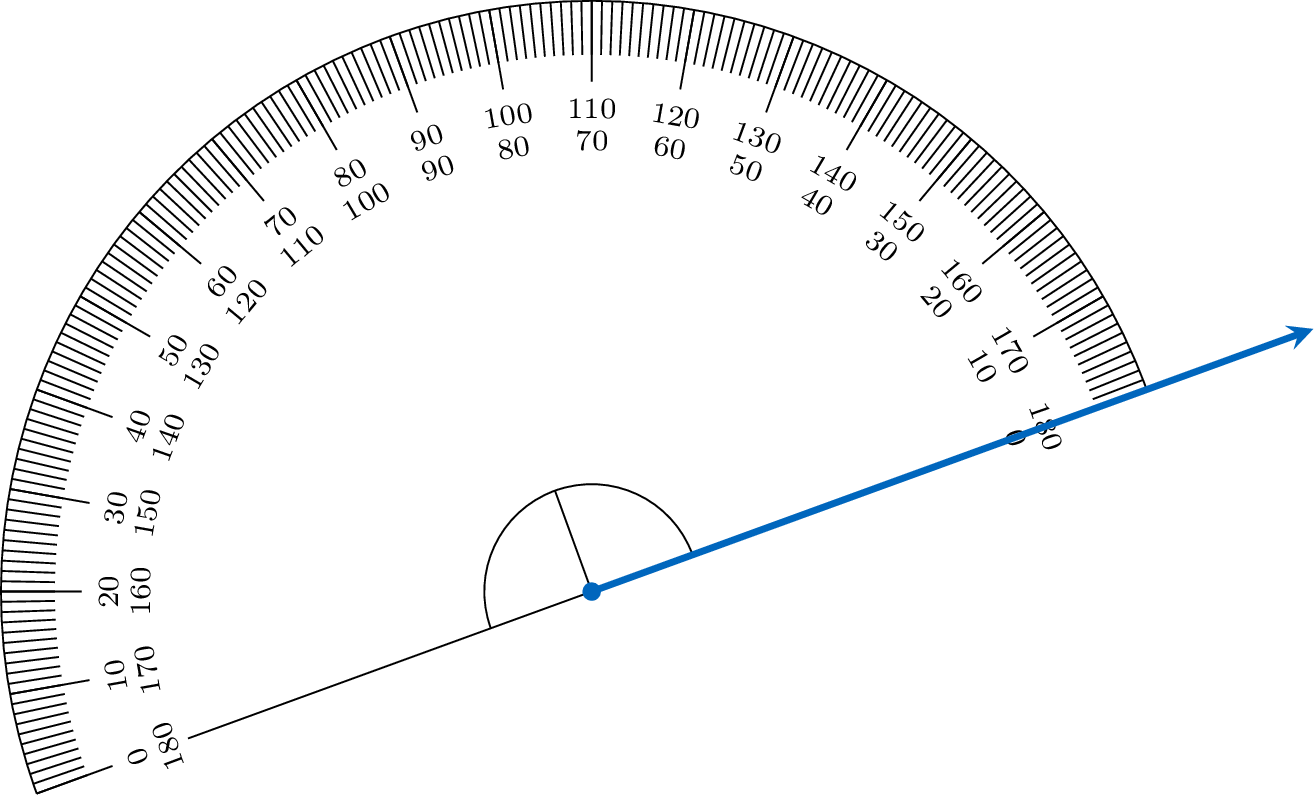
Locate the desired angle measure on the protractor’s scale and mark the point.
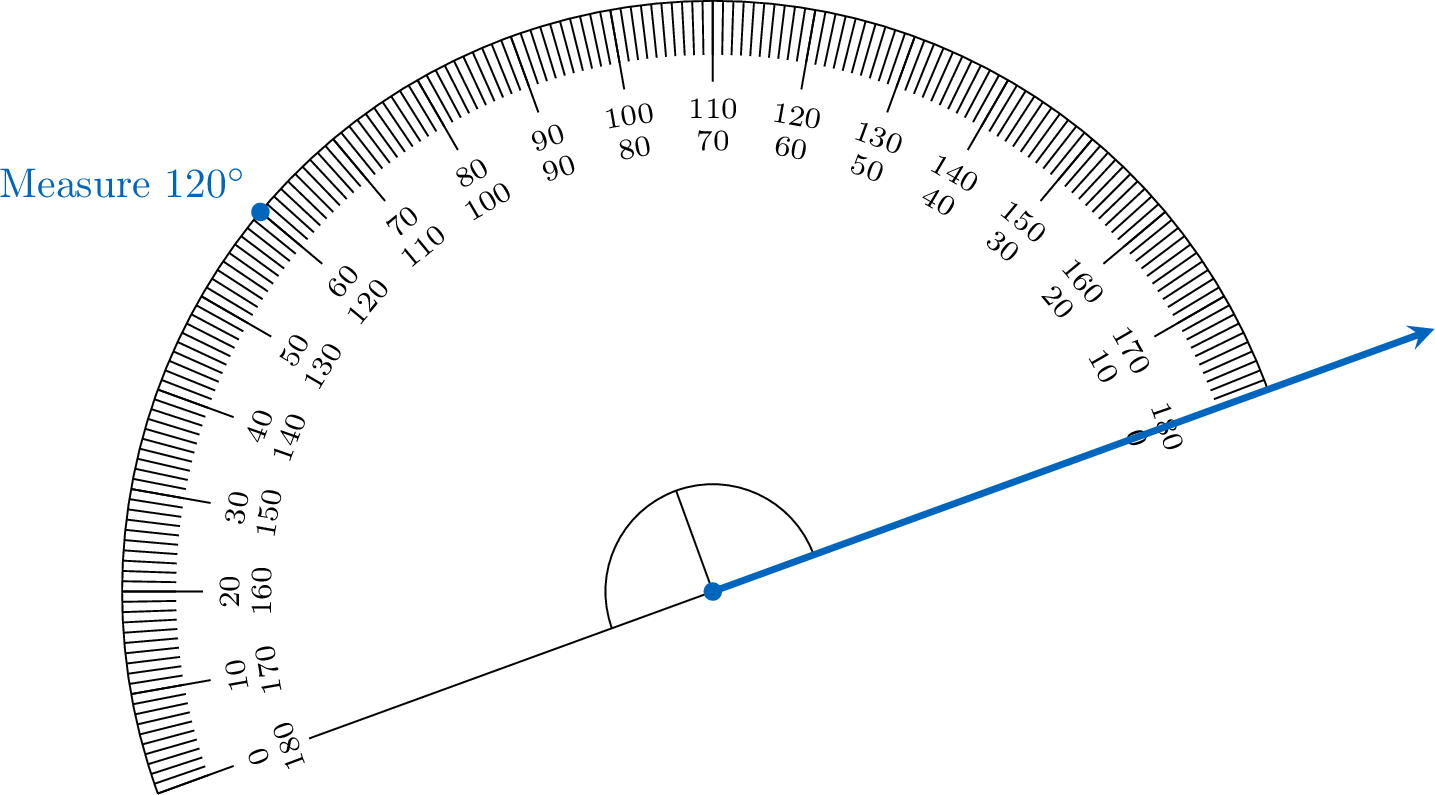
Draw a second ray from the vertex through the marked point to form the angle.

Classification of Angles

In geometry, angles are classified based on their measure. The following table defines four main types of angles: straight, right, acute, and obtuse.

Definition Classification of Angles

Name	Fraction of a Circle	Angle Measure	Figure
Acute Angle	Less than $\dfrac{1}{4}$	Less than $90^{\circ}$
Right Angle	$\dfrac{1}{4}$	$\dfrac{1}{4} \text{ of } 360^{\circ} = 90^{\circ}$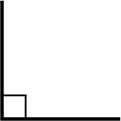
Obtuse Angle	Between $\dfrac{1}{4}$ and $\dfrac{1}{2}$	Between $90^{\circ}$ and $180^{\circ}$
Straight Angle	$\dfrac{1}{2}$	$\dfrac{1}{2} \text{ of } 360^{\circ} = 180^{\circ}$

Angle Addition

Proposition Angle Addition Postulate

When two angles share a common side and vertex, the measure of the angle they form is the sum of their measures.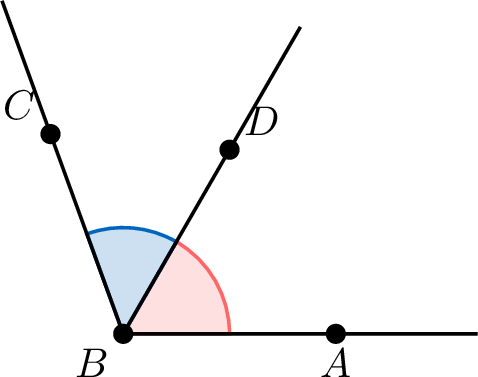

$$\Angle{ABC} = \textcolor{colordef}{\Angle{ABD}} + \textcolor{colorprop}{\Angle{DBC}}$$

Method Calculating an Angle

To find the measure of an unknown angle, use the measures of related known angles. If the unknown angle is formed by two smaller angles sharing a common side, add the measures of the smaller angles using the angle addition postulate.

Example

Calculate $\Angle{ABC}$ without a protractor.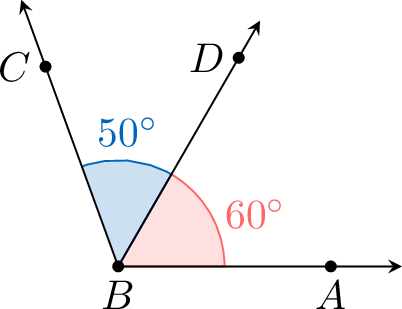

Answer

Using the angle addition postulate:$$\begin{aligned}\Angle{ABC} &= \textcolor{colordef}{\Angle{ABD}} + \textcolor{colorprop}{\Angle{DBC}} \\&= \textcolor{colordef}{ 60^\circ} + \textcolor{colorprop}{50^\circ} \\&= 110^\circ\end{aligned}$$

Angle Properties

Proposition Angle Properties

Right Angle: Adjacent angles that form a right angle have measures that sum to $90^\circ$.
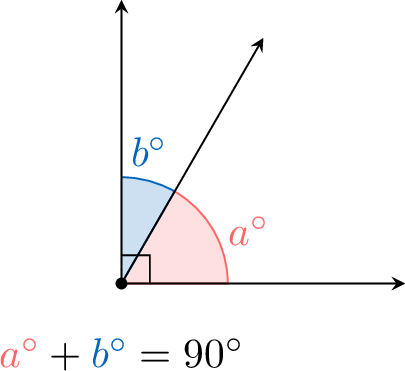
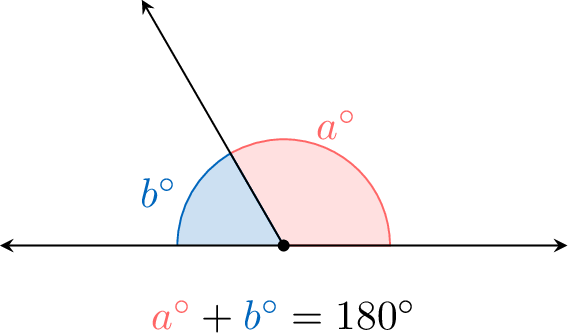
Straight Angle: Adjacent angles that form a straight angle have measures that sum to $180^\circ$.
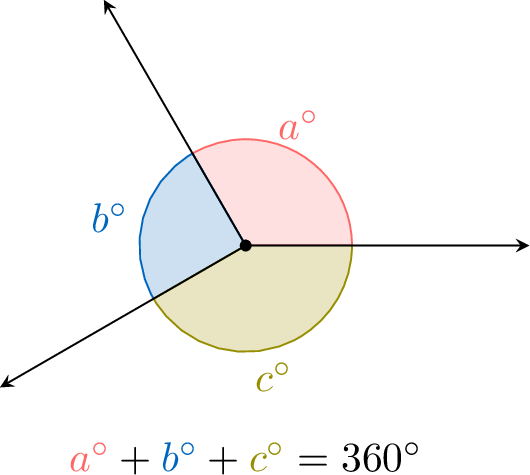
Full Angle: Angles around a point that form a complete rotation have measures that sum to $360^\circ$.

Example

Calculate $x^{\circ}$.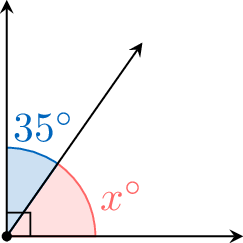

Answer

Since the angles form a right angle, their measures sum to $90^\circ$:$$\begin{aligned}x^\circ + 35^\circ &= 90^\circ \\x^\circ &= 90^\circ - 35^\circ \\&= 55^\circ\end{aligned}$$