Set Theory
Definitions
Definition Set
A set is a collection of objects, called elements.
We list its elements between curly brackets.
We list its elements between curly brackets.
Example
List all possible results when rolling a standard die 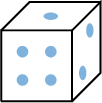 .
.
\(E=\{1,2,3,4,5,6\}=\{\) ,
,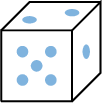 ,
,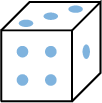 ,
,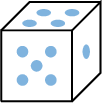 ,
,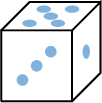 ,
, \(\}\).
\(\}\).
Definition Element
- An element is an object contained in a set.
- \(\in\) means "is an element of" or "belongs to".
- \(\notin\) means "is not an element of" or "does not belong to".
Example
\(2\in \{1,2,3,4,5,6\}\) and \(7\notin \{1,2,3,4,5,6\}\).
Definition Equal sets
Two sets are equal if they have exactly the same elements.
Example
Determine if the sets \(\{2,6,4\}\) and \(\{2,4,6\}\) are equal.
Yes, the sets \(\{2,6,4\}\) and \(\{2,4,6\}\) are equal because they contain the same elements: \(2\), \(4\), and \(6\).
Example
Determine if the sets \(\{1,2,3\}\) and \(\{1,2,4\}\) are equal.
No, the sets \(\{1,2,3\}\) and \(\{1,2,4\}\) are not equal because element \(3\) belongs to \(\{1,2,3\}\) but not to \(\{1,2,4\}\).
Ordered Pair
Definition Ordered Pair
An ordered pair, denoted \((a, b)\) or \(ab\), is a pair of objects in which their order is significant. The ordered pair \((1, 2)\) is different from the ordered pair \((2, 1)\).
Example
In a sprint relay race, two runners are paired up. Let \(L\) be Louis and \(H\) be Hugo. The ordered pair \((L, H)\) means Louis runs first, then passes the baton to Hugo. The ordered pair \((H, L)\) means Hugo runs first, then passes to Louis. These are different races.
Cardinality
Definition Cardinality
\(\Card{A}\) denotes the number of elements in the set \(A\).
Example
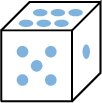
\(\Card{\{1,2,3,4,5,6\}}=6=\) 

Complement
Definition Universal set
A universal set is the set of all elements considered.
Definition Complement
The complement of a set \(A\), denoted \(A'\), consists of all elements in \(U\) that are not in \(A\). Sets \(A\) and \(A'\) are said to be complementary.
Example
Given the universe \(U = \{1, 2, 3, 4, 5, 6\}\) and the set \(A = \{1, 3, 5\}\), find the complement \(A'\).
Start with the universe \(U = \{1, 2, 3, 4, 5, 6\}\).
The set \(A = \{1, 3, 5\}\) includes 1, 3, and 5.
The complement \(A'\) is all the elements in \(U\) that are not in \(A\):
$$A' = \{2, 4, 6\}$$
The set \(A = \{1, 3, 5\}\) includes 1, 3, and 5.
The complement \(A'\) is all the elements in \(U\) that are not in \(A\):
$$A' = \{2, 4, 6\}$$