Probability
Ever wondered if it'll rain tomorrow or if you'll win a game? That's probability! It's a math way to guess how likely things are to happen. Like when the weather app says there's a 90\(\pourcent\) chance of rain, that's probability telling us it's very likely. What else do you think we could use probability for?
Algebra of Events
Sample Space
Have you ever flipped a coin and wondered if it’d land on heads or tails? Or rolled a die and guessed what number you’d get? These are random experiments—things we do where the result isn’t certain until it happens.
Definition Outcome
An outcome is one possible result of a random experiment.
Definition Sample Space
The sample space is the list of all possible outcomes of a random experiment.
Example
What’s the sample space when you flip a coin?
It’s \(\{\)Heads, Tails\(\}=\{\) ,
, \(\}\), or just \(\{\)H, T\(\}\) for short.
\(\}\), or just \(\{\)H, T\(\}\) for short.
Example
What’s the sample space when you roll a six-sided die?
It’s \(\{\)1, 2, 3, 4, 5, 6\(\}=\{\)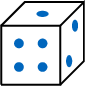 ,
,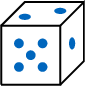 ,
,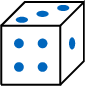 ,
,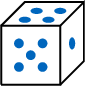 ,
,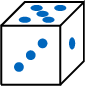 ,
, 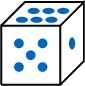 \(\}\).
\(\}\).
Events
Definition Event
An event is a set of outcomes from all possible outcomes.
In math, we use capital letters like \(E\) to name events. So, we might say \(E\) is the event “it’s sunny tomorrow.”
Example
You roll a die. Let \(E\) be the event of rolling an even number. What’s \(E\)?
The sample space is \(\{1, 2, 3, 4, 5, 6\}\), and \(E = \{2, 4, 6\}\) because those are the even numbers.
Complementary Event
Definition Complementary Event
The complementary event of an event \(E\) is everything in the sample space that isn’t in \(E\). We call it \(E'\) (\(E\)-prime).
Example
You roll a die, and \(E\) is rolling an even number. What’s \(E'\)?
\(E = \{2, 4, 6\}\), so \(E'\) is all the other numbers: \(E' = \{1, 3, 5\}\). These are the odd numbers!
Multi-Step Random Experiments
A multi-step random experiment is one that involves a sequence of actions, where each action (or step) has its own set of possible outcomes. In our example of tossing two coins, the experiment is multi-step because it involves two separate coin tosses:
- The first coin toss (step 1) can result in Heads (H) or Tails (T).
- The second coin toss (step 2) can also result in Heads (H) or Tails (T).
Method Representations of Multi-Step Random Experiment
When an experiment involves more than one step, we can represent the sample space (the set of all possible outcomes) in several ways:
- using a grid (to visually map combinations along two axes),
- using a table (to organize outcomes in rows and columns),
- using a tree diagram (to follow each sequential step), or
- by listing all possible outcomes.
Example
For the random experiment of tossing two coins, display the sample space by:
- using a grid
- using a table
- using a tree diagram
- listing all possible outcomes
-
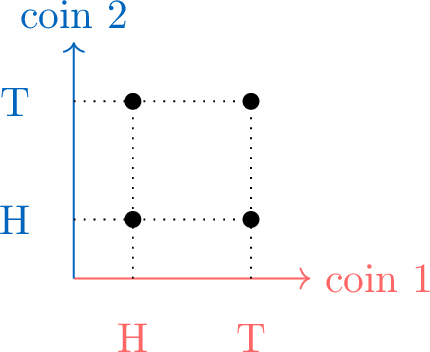
-
\(\begin{aligned} & \textcolor{colorprop}{\text{coin 2}} \\ \textcolor{colordef}{\text{coin 1}} \end{aligned} \) \(\textcolor{colorprop}{H}\) \(\textcolor{colorprop}{T}\) \(\textcolor{colordef}{H}\) \(\textcolor{colordef}{H}\textcolor{colorprop}{H}\) \(\textcolor{colordef}{H}\textcolor{colorprop}{T}\) \(\textcolor{colordef}{T}\) \(\textcolor{colordef}{T}\textcolor{colorprop}{H}\) \(\textcolor{colordef}{T}\textcolor{colorprop}{T}\) -
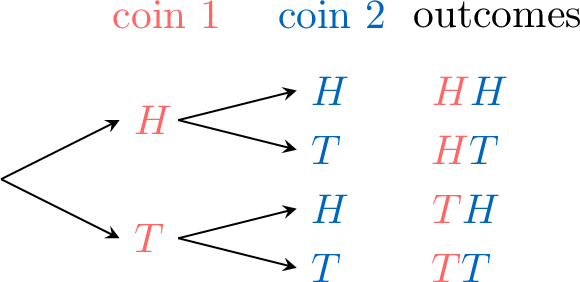
- \(\{\textcolor{colordef}{H}\textcolor{colorprop}{H}, \textcolor{colordef}{H}\textcolor{colorprop}{T},\textcolor{colordef}{T}\textcolor{colorprop}{H}, \textcolor{colordef}{T}\textcolor{colorprop}{T}\}\)
\(E\) or \(F\)
Definition \(E\) or \(F\)
The union of two events \(E\) and \(F\), denoted as \(E \text{ or } F\) or \(E \cup F\), is the event that occurs if either event \(E\) happens, event \(F\) happens, or both events happen simultaneously.
Example
Consider the roll of a standard six-sided die.
Let event \(E\) be the event of rolling an even number. So, \(E = \{2, 4, 6\}\).
Let event \(F\) be the event of rolling a number less than 4. So, \(F = \{1, 2, 3\}\).
Find the event \(E\) or \(F\).
Let event \(E\) be the event of rolling an even number. So, \(E = \{2, 4, 6\}\).
Let event \(F\) be the event of rolling a number less than 4. So, \(F = \{1, 2, 3\}\).
Find the event \(E\) or \(F\).
\(E\) or \(F\) includes all outcomes from both event sets.
So, \(E \text{ or } F = E \cup F = \{1, 2, 3, 4, 6\}\).
So, \(E \text{ or } F = E \cup F = \{1, 2, 3, 4, 6\}\).
\(E\) and \(F\)
Definition \(E\) and \(F\)
$$$$The intersection of two events \(E\) and \(F\), denoted as \(E \text{ and } F\) or \(E \cap F\), is the set of all outcomes that are common to both \(E\) and \(F\). The event \(E\) and \(F\) occurs if and only if both \(E\) and \(F\) happen simultaneously.
Example
Consider the roll of a standard six-sided die.
Let event \(E\) be the event of rolling an odd number. So, \(E = \{1, 3, 5\}\).
Let event \(F\) be the event of rolling a number less than 4. So, \(F = \{1, 2, 3\}\).
Find the event \(E\) and \(F\).
Let event \(E\) be the event of rolling an odd number. So, \(E = \{1, 3, 5\}\).
Let event \(F\) be the event of rolling a number less than 4. So, \(F = \{1, 2, 3\}\).
Find the event \(E\) and \(F\).
\(E\) and \(F\) includes all outcomes that are common to both \(E\) and \(F\).
So, \(E \text{ and } F = E \cap F = \{1, 3\}\).
So, \(E \text{ and } F = E \cap F = \{1, 3\}\).
Mutually Exclusive
Definition Mutually Exclusive
Two events \(E\) and \(F\) are said to be mutually exclusive if they cannot occur simultaneously. In other words, the occurrence of event \(E\) excludes the possibility of event \(F\) occurring, and vice versa. This is mathematically represented as:$$E \text{ and } F = E \cap F = \emptyset,$$where \(\emptyset\) denotes the empty set, indicating that there are no common outcomes between \(E\) and \(F\).
Example
Consider the roll of a standard six-sided die.
Let event \(E\) be the event of rolling an odd number. So, \(E = \{1, 3, 5\}\).
Let event \(F\) be the event of rolling an even number. So, \(F = \{2, 4, 6\}\).
Show that \(E\) and \(F\) are mutually exclusive.
Let event \(E\) be the event of rolling an odd number. So, \(E = \{1, 3, 5\}\).
Let event \(F\) be the event of rolling an even number. So, \(F = \{2, 4, 6\}\).
Show that \(E\) and \(F\) are mutually exclusive.
Since there are no common outcomes between \(E\) and \(F\), we have \(E \text{ and } F = E \cap F = \emptyset\). Thus, the events \(E\) and \(F\) are mutually exclusive.
Venn Diagram
Definition Correspondence Between Set Theory and Probabilistic Vocabulary
| Notation | Set Vocabulary | Probabilistic Vocabulary | Venn Diagram |
| \(U\) | Universal set | Sample space | 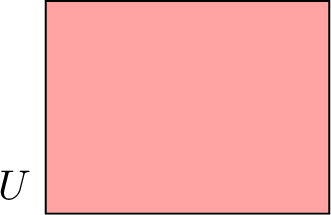 |
| \(x\) | Element of \(U\) | Outcome | 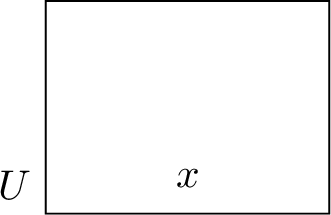 |
| \(\emptyset\) | Empty set | Impossible event | |
| \(E\) | Subset of \(U\) | Event | 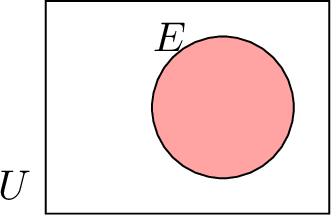 |
| \(x \in E\) | \(x\) is an element of \(E\) | \(x\) is an outcome of \(E\) | 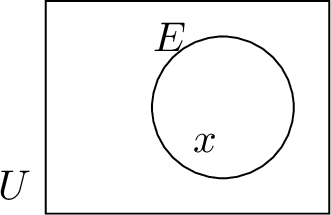 |
| \(E'\) | Complement of \(E\) in \(U\) | Complement of \(E\) in \(U\) | 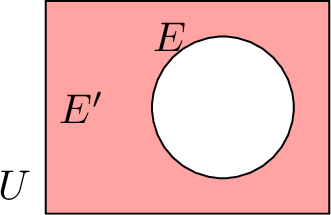 |
| \(E \text{ or } F\) | Union of \(E\) and \(F\): \(E \cup F\) | \(E\) or \(F\) | 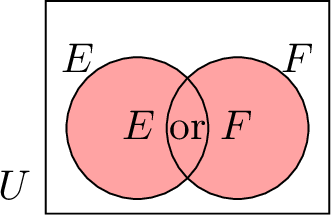 |
| \(E \text{ and } F\) | Intersection of \(E\) and \(F\): \(E \cap F\) | \(E\) and \(F\) | 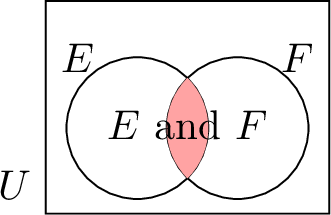 |
| \(E \cap F = \emptyset\) | \(E\) and \(F\) are disjoint | \(E\) and \(F\) are mutually exclusive | 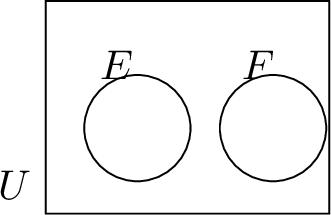 |
Probability
Probability Axioms
The probability of an event \(E\), denoted by \(P(E)\), is a value between 0 and 1 that represents the chance or likelihood of that event occurring.
We can visualize probabilities on a number line: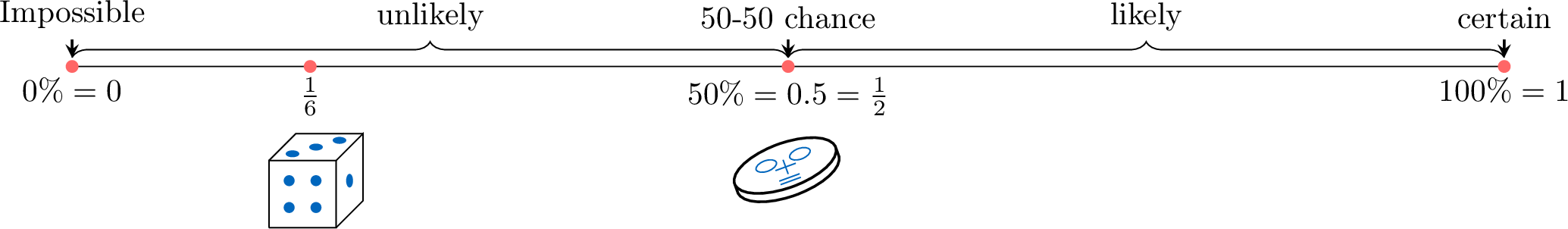
- The probability of an impossible event is \(0\) or \(0\pourcent\).
- The probability of a certain event is \(1\) or \(100\pourcent\).
- The probability of any event is between \(0\) and \(1\), inclusive.
We can visualize probabilities on a number line:
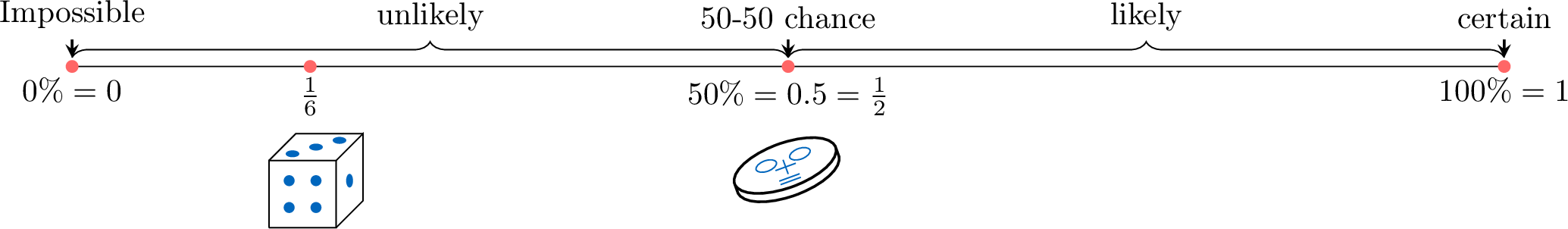
Definition Probability Axioms
\(P\) is a probability if:
- \(0 \leqslant P(E) \leqslant 1\), for any event \(E\),
- \(P(U) = 1\),
- If \(E\) and \(F\) are mutually exclusive events, then \(P(E \text{ or } F) = P(E) + P(F)\).
- The probability of an event \(E\), \(\textcolor{colordef}{P(E)}\), is represented by the shaded area of the event in a Venn diagram:

- The first axiom states that the probability of an event \(E\) is a value between 0 and 1, inclusive.
- The second axiom states that the probability of the sample space \(U\) is equal to \(1\), i.e., \(100\pourcent\). This is because the sample space \(U\) includes all possible outcomes of a random experiment, so the event \(U\) always occurs, and \(P(U) = 1\). In a Venn diagram, this is represented as the entire shaded area of the sample space:
 $$\textcolor{colordef}{P(U)} = 1$$
$$\textcolor{colordef}{P(U)} = 1$$ - The third axiom states that if two events are mutually exclusive (i.e., they cannot occur simultaneously), then the probability of their union is the sum of their individual probabilities. In a Venn diagram, for two mutually exclusive events (with no overlap), the total area of their union is the sum of the individual areas:
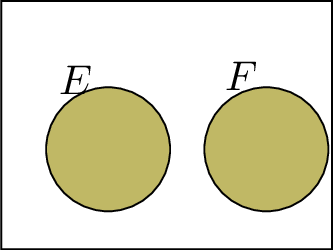 =
=
Probability Rules
Proposition Complement Rule
For any event \(E\) with complementary event \(E'\),$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = 1$$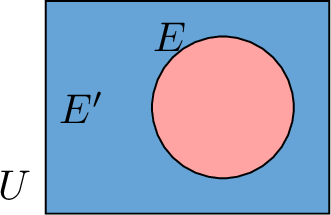

The green area, \(\textcolor{olive}{P(U)}\), ,is the sum of the red area, \(P(E)\), and the blue area, \(P(E')\),
,is the sum of the red area, \(P(E)\), and the blue area, \(P(E')\),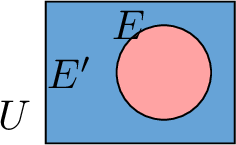 .So,$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = \textcolor{olive}{P(U)}$$Since \(\textcolor{olive}{P(U) = 1}\), we have:$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = 1$$
.So,$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = \textcolor{olive}{P(U)}$$Since \(\textcolor{olive}{P(U) = 1}\), we have:$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = 1$$
 ,is the sum of the red area, \(P(E)\), and the blue area, \(P(E')\),
,is the sum of the red area, \(P(E)\), and the blue area, \(P(E')\), .So,$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = \textcolor{olive}{P(U)}$$Since \(\textcolor{olive}{P(U) = 1}\), we have:$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = 1$$
.So,$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = \textcolor{olive}{P(U)}$$Since \(\textcolor{olive}{P(U) = 1}\), we have:$$\textcolor{colordef}{P(E)} + \textcolor{colorprop}{P(E')} = 1$$
Example
Farid has a 0.8 (80\(\pourcent\)) chance of finishing his homework on time tonight (event \(E\)). What’s the chance he doesn’t finish on time ?
The complementary event \(E'\) represents the scenario where Farid does not complete his homework on time tonight. As \(P(E) = 0.8\), by the complement rule, we get:$$\begin{aligned}P(E') &= 1 - 0.8 \\&= 0.2\end{aligned}$$So, there’s a 20 \(\pourcent\) chance he doesn’t finish on time!
Proposition Addition Law of Probability
For any events \(E\) and \(F\),$$P(E \text{ or } F) = P(E) + P(F) - P(E \text{ and } F)$$
Example
A local high school is holding a talent show where students can participate in singing, dancing, or both. The probability that a randomly selected student participates in singing is 0.4, the probability that a student participates in dancing is 0.3, and the probability that a student participates in both singing and dancing is 0.1. Find the probability that a randomly selected student participates in either singing or dancing.
- Let \(S\) be the event that a student participates in singing, and \(D\) be the event that a student participates in dancing. We are given \(P(S) = 0.4\), \(P(D) = 0.3\), and \(P(S \text{ and } D) = 0.1\).
- The probability of either singing or dancing is given by \(P(S \text{ or } D)\).
- By the addition law of probability,$$\begin{aligned}P(S \text{ or } D) &= P(S) + P(D) - P(S \text{ and } D) \\&= 0.4 + 0.3 - 0.1 \\&= 0.6\end{aligned}$$
- Thus, the probability that a student participates in either singing or dancing is \(0.6\).
Equally Likely
Sometimes, every outcome in an experiment has the same chance—like flipping a fair coin or rolling a fair die. We call these equally likely outcomes.
Definition Equally Likely
If all outcomes are equally likely, the probability of an event, \(E\), is given by $$P(E) = \frac{\text{number of outcomes in the event}}{\text{total number of all possible outcomes}}$$
Example
What’s the probability of rolling an even number with a fair six-sided die?
- Sample space = \(\{1, 2, 3, 4, 5, 6\}\) (6 outcomes).
- \(E = \{2, 4, 6\}\) (3 outcomes).
- $$\begin{aligned}P(E) &= \frac{3}{6}\\&= \frac{1}{2}\end{aligned}$$.
Probability of Independent Events
Independent events are like you choosing an ice cream flavor and your friend picking a movie—your choice doesn’t affect theirs, and theirs doesn’t affect yours! For example, flipping a coin and rolling a die at the same time: whether you get heads or tails has no effect on what number you roll. Let’s explore this idea together!
Definition Independent Events
Two events, \(A\) and \(B\), are independent if the chance of both happening is just the product of their individual chances. Mathematically:$$P(A \text{ and } B) = P(A) \times P(B)$$
Example
Imagine you do two totally separate actions:
- Flipping a fair coin (heads or tails).
- Rolling a fair six-sided die (1, 2, 3, 4, 5, or 6).
Let’s break it down:
- These events are independent, so we multiply their probabilities.
- For the coin: You’ve got two options—heads or tails—and they’re equally likely. So, \(P(\text{"tails"}) = \dfrac{1}{2}\).
- For the die: There are six sides, and “greater than 4” means 5 or 6. That’s 2 out of 6 possibilities, so \(P("\text{number} > 4") = \dfrac{2}{6}=\dfrac{1}{3}\).
- Now, combine them: $$\begin{aligned} P(\text{"tails" and "number} > 4") &= P(\text{"tails"}) \times P(\text{"number} > 4") \\ &= \dfrac{1}{2} \times \dfrac{1}{3} \\ &= \dfrac{1}{6} \end{aligned}$$
- Result: There’s a \(\dfrac{1}{6}\) chance of landing tails and rolling a 5 or 6.
Method Finding the probability of two independent events using a probability tree diagram
Let’s use a probability tree for our coin flip and die roll:
- Draw the Tree: Start with two branches for the coin: “Heads” and “Tails.” Then, from each, draw two more branches for the die: “Number > 4” (5 or 6) and “Number \(\leqslant\) 4” (1, 2, 3, or 4).
- Add Probabilities: Label each branch. The coin gives \(\dfrac{1}{2}\) for “Tails” and \(\dfrac{1}{2}\) for “Heads.” For the die, “Number > 4” is \(\dfrac{1}{3}\) (2 out of 6), and “Number \(\leqslant\) 4” is \(\dfrac{2}{3}\) (4 out of 6).
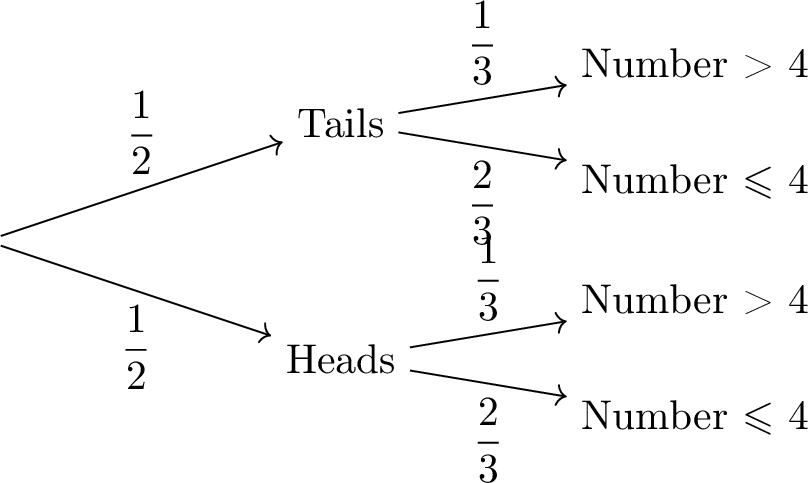
- Follow the Path: To find \(P(\text{tails and number} > 4)\), trace the “Tails” branch, then the “Number > 4” branch, and multiply: $$\dfrac{1}{2} \times \dfrac{1}{3} = \dfrac{1}{6}$$
- Wrap It Up: The tree confirms our answer—\(\dfrac{1}{6}\)!
Experimental Probability
Isaac wants to know how a cone lands when he tosses it—base down or point down? Here’s what it can do:
- Base down:
- Point down:
- Base down: 30 times.
- Point down: 20 times.
- \( P(\text{"base down"}) = \frac{30}{50} = 0.6 \) (60\(\pourcent\)).
- \( P(\text{"point down"}) = \frac{20}{50} = 0.4 \) (40\(\pourcent\)).
Definition Experimental Probability
The probability \( P(E) \) of an event \( E \) can be estimated using the formula:$$P(E) \approx \frac{\text{number of times the event occurs}}{\text{number of trials}}$$
Expected Value
Suppose a fair six-sided die is rolled 120 times. Find the expected number of times the result will be a "six" =  ,.
,.
The sample space when rolling a fair six-sided die is \(\{1, 2, 3, 4, 5, 6\}\), with each outcome equally likely. Thus, the probability of rolling a "six" in a single trial is:$$P(\text{six}) = \frac{1}{6}$$Since the die is rolled 120 times, the expected number of "sixes" is:$$120 \times \frac{1}{6} = 20$$So, we expect 20 of the 120 rolls to result in a "six."
Proposition Expected Value
When an experiment is performed \(n\) times (called trials) and the probability of an event occurring in each trial is \(p\), the expected value (or expectation) of the number of occurrences of that event is:$$n \times p$$This represents the average number of times the event is expected to occur over \(n\) trials.
Example
Jane enters a raffle where the chance of winning a prize with each ticket is \(\frac{1}{10}\). She buys 20 raffle tickets. Find the expected number of prizes Jane is likely to win.
Let’s calculate the expected value:
- The probability of winning with one ticket is:$$p = P(\text{"winning with a ticket"}) = \frac{1}{10}$$
- The number of trials (tickets) is:$$n = 20$$
- The expected number of prizes is:$$\begin{aligned}n \times p &= 20 \times \frac{1}{10} \\&= \frac{20}{10} \\&= 2\end{aligned}$$