Division with Remainders
Division with a remainder is a way of dividing when you don't have enough to make equal groups. It's like sharing things, and sometimes there's a little bit left over.
A) Division without Remainders
Definition Division
Division is
- splitting a total into equal groups:
- regrouping a total into groups of equal size:
Example
Hugo has 
How many marbles are in each group?
There are
B) Division with Remainders
Let's look at our marble example again. Now, Hugo has 
How many marbles are in each group? And how many are left over?

There are
Definition Division with remainder
When you divide one number by another, sometimes there is something left over. The number that's left over is called the remainder. 
C) Long Division
- To divide
- Think of the multiplication problem:
- Find how many times
- Answer:
- Think of the multiplication problem:
- To divide with a remainder, like
- Think of the multiplication problem:
- Find how many times
-
-
-
- Calculate the difference:
- Answer:
- Think of the multiplication problem:
Method Column Division 1 Step
To divide with a remainder, like
-
Set up the division problem -
How many times does Write -
Subtract -
Method Column Division 2 Steps
For the division with a remainder of
-
Set up the division problem -
How many times does -
Subtract -
How many times does -
Subtract: -
D) Two Ways to Think About Division
Method Finding number in each group and remainder
If we know the 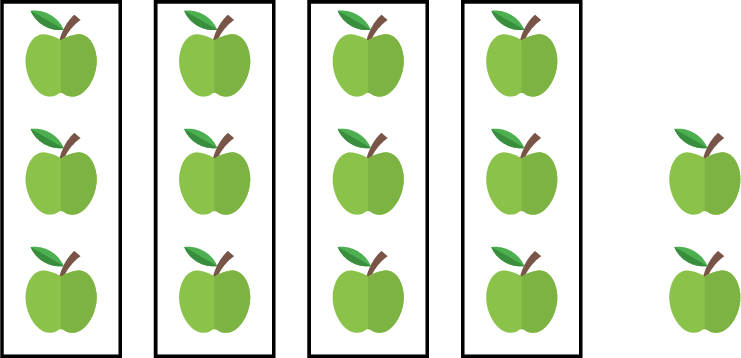
Each friend gets
There are

Each friend gets
There are
Method Finding number of groups and remainder
If we know the 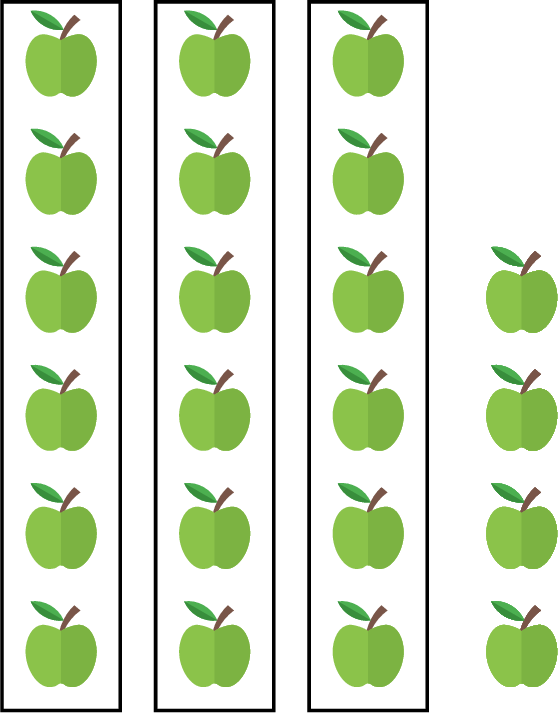
We pack
There are

We pack
There are