5-digit numbers
Definitions
- When we have 10 ones=
 , we group them into 1 ten=
, we group them into 1 ten= .
. - When we have 10 tens=
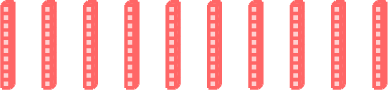 , we group them into 1 hundred=
, we group them into 1 hundred= .
. - When we have 10 hundreds =
 , we group them into 1 thousand =
, we group them into 1 thousand =
- When we have 10 thousands =
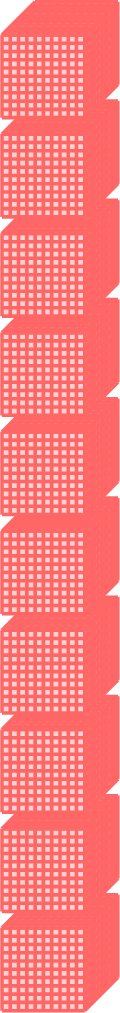 , we group them into 1 ten thousand=
, we group them into 1 ten thousand=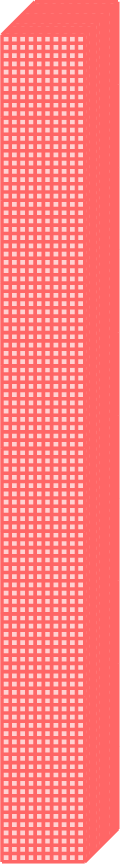
- To count how many ten thousands, thousands, hundreds, tens, and ones there are, we can make a table:
Ten Thousands Thousands Hundreds Tens Ones 1 2 1 2 3 




Definition Base 10 system
In the base 10 system, the place of a digit in a number determines its value. We can represent the number \(32\,354\) in several ways:
- With digits: $$32\,354$$
- In expanded form:$$\begin{aligned}[t]3\text{ ten-thousands } &+ &2\text{ thousands } &+ &3\text{ hundreds } &+ &5\text{ tens } &+ &4\text{ ones }\\30\,000 &+ &2\,000 &+ &300 &+ &50 &+ &4 \\3\times 10\,000&+& 2\times 1\,000&+& 3\times 100&+&5\times 10&+&4\times 1\\\end{aligned}$$
- With words:
thirty-two thousand three hundred fifty-four - In a table:
Ten-Thousands Thousands Hundreds Tens Ones 3 2 3 5 4 - With cubes:

On the Number Line
- A number line shows numbers like 0, 1, 2, 3, and so on in order.
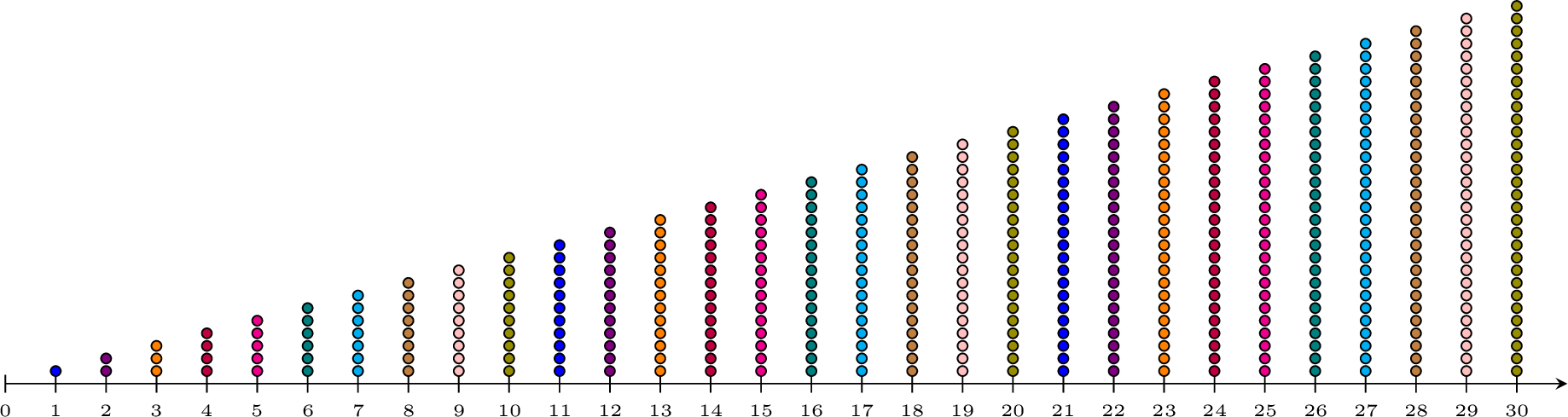
- Let’s make counting easier by counting by tens on our number line. Now we jump 10 at a time: 0, 10, 20, 30.

Definition Number Line
A number line is a line that shows numbers in order. Moving right adds by same number.
