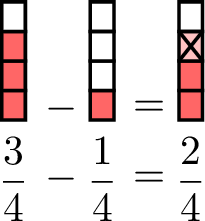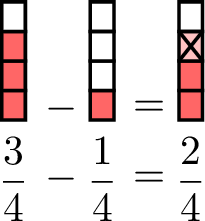Fractions
Definitions
Hugo is very hungry after playing soccer. His dad baked two identical cakes.
Hugo eats one whole cake:

Hugo eats one whole cake:
- Hugo eats one whole cake and half of another cake.

- The numerator (top number) shows how many parts Hugo eats: \(3\).
- The denominator (bottom number) shows how many equal parts make one cake: \(2\).
- So Hugo eats \(\dfrac{3}{2}\) cakes in total.
Definition Fraction
A fraction includes two numbers: the numerator and the denominator, separated by a bar. 

On the number line
- Hugo is walking along a path.

- He stops and asks himself, "Where am I?"
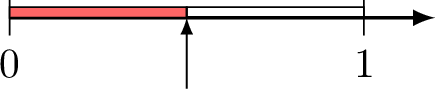
- His father says, "You are at half of the way that is \(\dfrac{1}{2}\)."
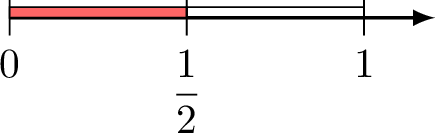
Method Representing a Fraction on the Number Line
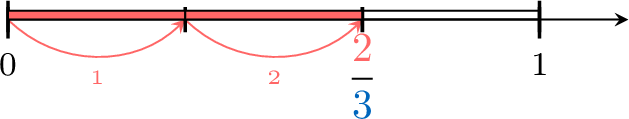
To represent the fraction \(\dfrac{\textcolor{colordef}{2}}{\textcolor{colorprop}{3}}\) on a number line.
- Draw a straight line and mark the points 0 and 1.

- Divide the line between 0 and 1 into \(\textcolor{colorprop}{3}\) equal parts.

- Count \(\textcolor{colordef}{2}\) parts from 0 and mark the point.
Equivalent Fractions
Mr. Tariel has a cake that he cuts into 3 equal parts. He plans to give 1 part to his son, Louis.
His dad replies, "Alright," and cuts each of the 3 parts in half, making 6 smaller equal parts. He then gives Louis 2 of these smaller pieces.
Why is Louis still not happy?
His dad replies, "Alright," and cuts each of the 3 parts in half, making 6 smaller equal parts. He then gives Louis 2 of these smaller pieces.
Why is Louis still not happy?
Even though Louis got 2 pieces instead of 1, the total amount of cake he received is the same as before. His dad just cut the cake into smaller pieces. \(\quad =\quad \)
\(\quad =\quad \)
Definition Equivalent Fractions
When you multiply the numerator and the denominator by the same number, the fractions are equals.
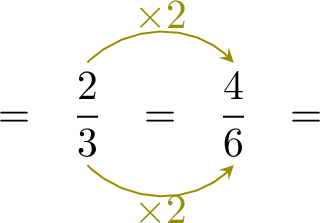

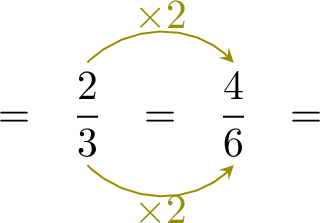
Addition and Subtraction
Hugo eats \(\dfrac{2}{4}\) of a cake:  and Louis eats \(\dfrac{1}{4}\) of the same cake:
and Louis eats \(\dfrac{1}{4}\) of the same cake: 
Which fraction of the cake have Hugo and Louis eaten together?
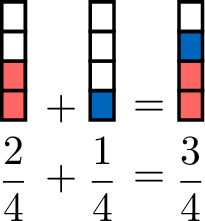

Which fraction of the cake have Hugo and Louis eaten together?
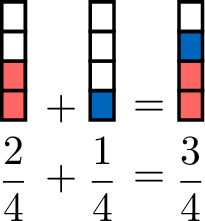
Definition Addition of Fractions with Common Denominators
When we add fractions with common denominators, we keep the denominator the same and add the numerators: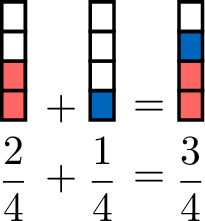
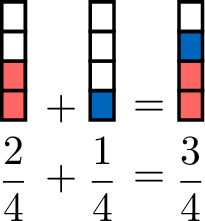
Definition Subtraction of Fractions with Common Denominators
When we subtract fractions with common denominators, we keep the denominator the same and subtract the numerators: