Fractions
Definitions
- Hugo shares a cake with his brother Louis: 1 cake =

- Hugo cuts the cake into two equal parts:

- Hugo takes 1 of 2 parts:

- His father asks, "Can you represent the part you have with a number?" Hugo thinks carefully. "I know numbers like 0, 1, 2, and so on. But 1 represents a whole cake, and 0 means no cake at all." Then he realizes, "I can use a fraction!" His father smiles and says, "Exactly! We write it as $$\dfrac{\textcolor{colordef}{1}}{\textcolor{colorprop}{2}}$$ where \(\textcolor{colordef}{1}\) is the number of parts you have, and \(\textcolor{colorprop}{2}\) is the total number of equal parts in the whole cake."
Definition Fraction
A fraction includes two numbers: the numerator and the denominator, separated by a bar. 

On the number line
- Hugo is walking along a path.

- He stops and asks himself, "Where am I?"
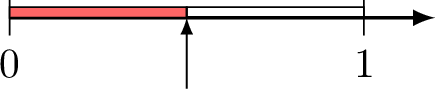
- His father says, "You are at half of the way that is \(\dfrac{1}{2}\)."
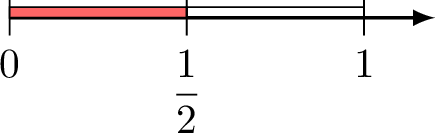
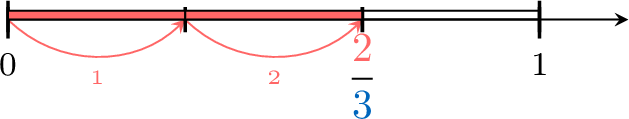
Method Representing a Fraction on the Number Line
To represent the fraction \(\dfrac{\textcolor{colordef}{2}}{\textcolor{colorprop}{3}}\) on a number line.
- Draw a straight line and mark the points 0 and 1.

- Divide the line between 0 and 1 into \(\textcolor{colorprop}{3}\) equal parts.

- Count \(\textcolor{colordef}{2}\) parts from 0 and mark the point.